Mechanical vibrations: what is it? Mechanical vibrations. Parameters of oscillatory motion What is needed to describe mechanical vibration

Time should be devoted to a short essay on oscillatory motion. But first, one important question needs to be answered. What is meant by mechanical vibrations? They mean movement during which the observed body repeatedly occupies the same positions in space.

Physicists distinguish between non-periodic and periodic oscillations. The former include those for which the coordinates and other characteristics of the body cannot be described using periodic functions of time. The second kind is easier. Periodic oscillations are those that can be described using periodic functions of time. But what do they mean? In physics, oscillations are also often understood as processes that are repeated to a certain extent in time. And separately regarding the topic under consideration, the following should be said. Mechanical vibrations conditionally can be classified as follows:

1. Depending on the conditions of occurrence:
1. Forced;
2. Self-oscillations;
3. Free.
2. Depending on the change in kinetic energy over time:
1. Harmonic;
2. sawtooth;
3. Decaying.

The article will not consider all, but only some types of oscillations. Separately, it is worth mentioning the formulas, their use and diversity. In short, there are many. The variety in which mechanical vibrations are presented, the formulas for determining their parameters prompted scientists to create separate reference books designed for certain situations. Invent yourself, so you don't need anything. When creating an oscillatory system, it will only take half an hour or an hour to find a formula for a specific situation.

Characteristics of mechanical vibrations

To characterize mechanical vibrations, physical quantities are used that allow obtaining the necessary data. Oscillation amplitude - the largest deviation of the body that oscillates from the initial position value. What is a period? In it, oscillations are the time it takes the body to repeat all its movements, or in other words, the time it takes to complete one repetition of a movement. What is meant by frequency? Under it understand the number equal to the number of oscillations made in one unit of time. Often in home, school and university experiments, one second is taken as the frequency. The cyclic frequency is often used instead of the concept of the number of oscillations that have occurred per unit of time, and implies its count required to complete one such cycle.

Harmonic mechanical vibrations

Harmonic vibrations are those that physical quantity which, selected for the characteristic, changes over the time interval in the form of a sinusoidal curve, which is easy to display in graphical mode. When the coordinate of a material point changes, according to the harmonic law, the momentum, speed and acceleration also change according to it.

Free vibrations

When an oscillation takes place in the system due to the initial energy, it is called free. As a practical representation of this type of physical process, special models are used: spring and mathematical pendulums. They allow you to work with the most common situations. As a mathematical pendulum, they take a point that oscillates and hangs on an inextensible and weightless thread. There is no such device on earth. Therefore, closest to the theoretical model is a structure made up of a ball, the diameter (size) of which is much smaller than the length of the thread. It is necessary to carry out actions of a physical nature. Deviate such a ball from its initial position and release. And so any experimenter will be able to see mechanical vibrations. The period, as well as their frequency, depend solely on the parameters of the system: the length of the thread of the mathematical pendulum, the stiffness of the spring, the mass of the load (important for a spring pendulum). It is because of this that free oscillations are also called natural oscillations of the system. Quite logical. And the frequency with which everything happens is called the system frequency.

Energy conversion during mechanical vibrations

Potential and kinetic energy during the movements of the body pass one into the other. And the same is the opposite. When the system deviates from the initial equilibrium position by the largest possible value, then the potential energy also reaches its maximum value, while the kinetics of the body reaches its minimum. Separately, it should be said about one misconception that is popular among people. When the equilibrium position is reached, then the potential energy is at its minimum (it is usually believed that here it is equal to zero), while the kinetics (and this is both the momentum of the body and the speed of its movement) reaches its maximum. In practice, something else is taken into account. V real systems there are non-potential forces, the value of which is not equal to zero. The energy of the system is wasted due to the work of the support forces, air friction, internal forces springs or suspension. The amplitude of body oscillation gradually decreases. Such oscillations are called damped. If the friction force is too great, then the entire energy reserve can be used up already during the period of one oscillation, and the motion of the body will not be periodic.

Forced vibrations

Forced oscillations are understood to mean those that occur under the influence of an external force that does work, which changes with time. There is another wording. Thanks to an external influx of energy, it is maintained in the system itself at a sufficient level for actual oscillations to occur. To understand this, it is necessary to draw parallels with reality. An example of an object that makes this type of oscillation is a swing on which one person sits, and the second one swings it. There is one nuance. If an external force compensates for the loss of energy in the system continuously or periodically, without stopping the oscillation process itself, then they are called undamped forced oscillations.

The following can be noted about the range. The amplitude of forced oscillations is completely determined by the force that acts from the outside, as well as the ratio between the natural frequencies of the parties involved in the process. And here an interesting phenomenon takes place. With forced oscillations, a sharp increase in amplitude can be periodically observed, which is called resonance.

Resonance

It occurs when the force that affects the system becomes very close to its oscillation frequency. Another option is also possible. In the event that the frequency of the influencing force is a multiple of the oscillations of the system itself, on which it acts, resonance also occurs. How is it graphically represented? The dependence of the oscillation amplitudes of the system on the frequency of the influencing force is expressed using a resonance curve.

Self-oscillations

Self-oscillations have found their application in technology. They exist where undamped oscillations are maintained by the energy of a source that the system itself can turn on and off automatically. In such cases, one can seriously consider the question of assigning the status of a self-oscillating system to the system. Why? The moment when it is necessary to supply energy for oscillation is monitored by the subsystem responsible for feedback. Depending on the parameters of the body, it can affect strongly and immediately, or little by little and gradually. It can open or close the opportunity for energy to enter common system. This is her main task. As an example of a self-oscillating system, one can recall a pendulum clock, where the energy source is a weight, and the anchor mechanism successfully copes with the role of a subsystem feedback, which regulates the supply of kinetics, on which mechanical vibrations depend.

Parametric vibrations

Under this type of oscillations are defined those that occur in systems that periodically change their parameters. What can be said about them? The only thing that determines the amplitude and strength of an oscillatory system is its parameters.

1. Fluctuations. periodic fluctuations. Harmonic vibrations.

2. Free vibrations. Undamped and damped oscillations.

3. Forced vibrations. Resonance.

4. Comparison of oscillatory processes. Energy of undamped harmonic oscillations.

5. Self-oscillations.

6. Oscillations of the human body and their registration.

7. Basic concepts and formulas.

8. Tasks.

1.1. Fluctuations. periodic fluctuations.

Harmonic vibrations

fluctuations processes that differ in varying degrees of repetition are called.

recurring processes continuously occur inside any living organism, for example: heart contractions, lung function; we shiver when we are cold; we hear and speak thanks to the vibrations of the eardrums and vocal cords; When walking, our legs make oscillatory movements. The atoms that make us vibrate. The world we live in is remarkably prone to fluctuations.

Depending on the physical nature of the repeating process, oscillations are distinguished: mechanical, electrical, etc. This lecture discusses mechanical vibrations.

Periodic fluctuations

periodic called such oscillations in which all the characteristics of the movement are repeated after a certain period of time.

For periodic oscillations, the following characteristics are used:

oscillation period T, equal to the time during which one complete oscillation takes place;

oscillation frequencyν, equal to the number of oscillations per second (ν = 1/T);

oscillation amplitude A, equal to the maximum displacement from the equilibrium position.

Harmonic vibrations

A special place among periodic fluctuations is occupied by harmonic fluctuations. Their importance is due to the following reasons. Firstly, oscillations in nature and technology often have a character very close to harmonic, and secondly, periodic processes of a different form (with a different time dependence) can be represented as a superposition of several harmonic oscillations.

Harmonic vibrations- these are oscillations in which the observed value changes in time according to the law of sine or cosine:

In mathematics, functions of this kind are called harmonic, therefore, oscillations described by such functions are also called harmonic.

The position of a body that performs an oscillatory motion is characterized by displacement about the equilibrium position. In this case, the quantities in formula (1.1) have the following meaning:

X- bias body at time t;

A - amplitude fluctuations equal to the maximum displacement;

ω - circular frequency oscillations (the number of oscillations made in 2 π seconds), related to the oscillation frequency by the ratio

φ = (ωt +φ 0) - phase fluctuations (at time t); φ 0 - initial phase oscillations (at t = 0).

Rice. 1.1. Plots of offset versus time for x(0) = A and x(0) = 0

1.2. Free vibrations. Undamped and damped oscillations

Free or own called such oscillations that occur in a system left to itself, after it has been taken out of equilibrium.

An example is the oscillation of a ball suspended on a thread. In order to cause vibrations, you need to either push the ball, or, moving it aside, release it. When pushed, the ball is informed kinetic energy, and in case of deviation - potential.

Free oscillations are performed due to the initial energy reserve.

Free undamped vibrations

Free oscillations can be undamped only in the absence of friction force. Otherwise, the initial supply of energy will be spent on overcoming it, and the range of oscillations will decrease.

As an example, consider the vibrations of a body suspended on a weightless spring, which occur after the body is deflected downward and then released (Fig. 1.2).

Rice. 1.2. Vibrations of a body on a spring

From the side of the stretched spring, the body acts elastic force F proportional to the amount of displacement X:

The constant factor k is called spring rate and depends on its size and material. The "-" sign indicates that the elastic force is always directed in the direction opposite to the displacement direction, i.e. to the equilibrium position.

In the absence of friction, the elastic force (1.4) is the only force acting on the body. According to Newton's second law (ma = F):

After transferring all the terms to the left side and dividing by the body mass (m), we obtain a differential equation for free oscillations in the absence of friction:

The value ω 0 (1.6) turned out to be equal to the cyclic frequency. This frequency is called own.

Thus, free vibrations in the absence of friction are harmonic if, when deviating from the equilibrium position, elastic force(1.4).

Own circular frequency is the main characteristic of free harmonic oscillations. This value depends only on the properties of the oscillating system (in the case under consideration, on the mass of the body and the stiffness of the spring). In what follows, the symbol ω 0 will always be used to denote own circular frequency(i.e., the frequency at which vibrations would occur in the absence of friction).

Amplitude of free vibrations is determined by the properties of the oscillatory system (m, k) and the energy imparted to it at the initial moment of time.

In the absence of friction, free oscillations close to harmonic ones also arise in other systems: mathematical and physical pendulums (the theory of these issues is not considered) (Fig. 1.3).

Mathematical pendulum- a small body (material point) suspended on a weightless thread (Fig. 1.3 a). If the thread is deflected from the equilibrium position by a small (up to 5°) angle α and released, then the body will oscillate with a period determined by the formula

where L is the length of the thread, g is the free fall acceleration.

Rice. 1.3. Mathematical pendulum (a), physical pendulum (b)

physical pendulum- solid, which oscillates under the action of gravity around a fixed horizontal axis. Figure 1.3 b schematically shows a physical pendulum in the form of a body of arbitrary shape, deviated from the equilibrium position by an angle α. The oscillation period of a physical pendulum is described by the formula

where J is the moment of inertia of the body about the axis, m is the mass, h is the distance between the center of gravity (point C) and the suspension axis (point O).

The moment of inertia is a quantity that depends on the mass of the body, its dimensions and position relative to the axis of rotation. The moment of inertia is calculated using special formulas.

Free damped vibrations

Friction forces acting in real systems significantly change the nature of motion: the energy of an oscillatory system constantly decreases, and oscillations either fade out or do not occur at all.

The resistance force is directed in the direction opposite to the movement of the body, and at not very high speeds it is proportional to the speed:

A graph of such fluctuations is shown in Fig. 1.4.

As a characteristic of the degree of attenuation, a dimensionless quantity is used, called logarithmic damping decrementλ.

Rice. 1.4. Displacement versus time for damped oscillations

Logarithmic damping decrement is equal to the natural logarithm of the ratio of the amplitude of the previous oscillation to the amplitude of the subsequent oscillation.

where i is the ordinal number of the oscillation.

It is easy to see that the logarithmic damping decrement is found by the formula

Strong attenuation. At

if the condition β ≥ ω 0 is fulfilled, the system returns to the equilibrium position without oscillating. Such a movement is called aperiodic. Figure 1.5 shows two possible ways return to the equilibrium position during aperiodic motion.

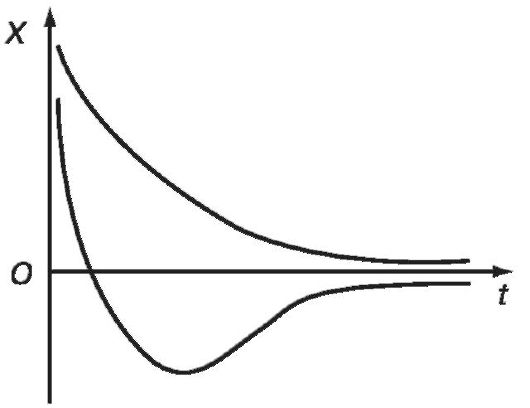Rice. 1.5. aperiodic motion

1.3. Forced vibrations, resonance

Free vibrations in the presence of friction forces are damped. Continuous oscillations can be created with the help of a periodic external action.

compelled such oscillations are called, during which the oscillating system is exposed to an external periodic force (it is called a driving force).

Let the driving force change according to the harmonic law

The graph of forced oscillations is shown in Fig. 1.6.

Rice. 1.6. Plot of displacement versus time for forced vibrations

It can be seen that the amplitude of forced oscillations reaches a steady value gradually. The steady forced oscillations are harmonic, and their frequency is equal to the frequency of the driving force:

The amplitude (A) of steady forced oscillations is found by the formula:

Resonance is called the achievement of the maximum amplitude of forced oscillations at a certain value of the frequency of the driving force.

If condition (1.18) is not satisfied, then resonance does not arise. In this case, as the frequency of the driving force increases, the amplitude of forced oscillations decreases monotonically, tending to zero.

The graphical dependence of the amplitude A of forced oscillations on the circular frequency of the driving force at different values ​​of the damping coefficient (β 1 > β 2 > β 3) is shown in fig. 1.7. Such a set of graphs is called resonance curves.

In some cases, a strong increase in the amplitude of oscillations at resonance is dangerous for the strength of the system. There are cases when resonance led to the destruction of structures.

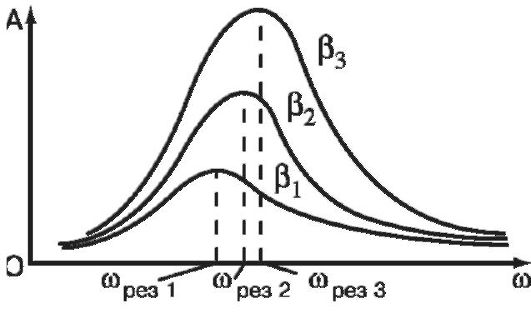Rice. 1.7. Resonance curves

1.4. Comparison of oscillatory processes. Energy of undamped harmonic oscillations

Table 1.1 presents the characteristics of the considered oscillatory processes.

Table 1.1. Characteristics of free and forced vibrations

Energy of undamped harmonic oscillations

A body that performs harmonic oscillations has two types of energy: the kinetic energy of motion E k \u003d mv 2 / 2 and the potential energy E p associated with the action of an elastic force. It is known that under the action of elastic force (1.4) the potential energy of the body is determined by the formula E p = kx 2 /2. For undamped oscillations X= A cos(ωt), and the speed of the body is determined by the formula v= - A ωsin(ωt). From this, expressions are obtained for the energies of a body performing undamped oscillations:

The total energy of the system in which undamped harmonic oscillations occur is the sum of these energies and remains unchanged:

Here m is the mass of the body, ω and A are the circular frequency and amplitude of oscillations, k is the coefficient of elasticity.

1.5. Self-oscillations

There are systems that themselves regulate the periodic replenishment of lost energy and therefore can fluctuate for a long time.

Self-oscillations- undamped oscillations supported by an external source of energy, the supply of which is regulated by the oscillatory system itself.

Systems in which such oscillations occur are called self-oscillating. The amplitude and frequency of self-oscillations depend on the properties of the self-oscillating system itself. The self-oscillatory system can be represented by the following scheme:

In this case, the oscillatory system itself, through a feedback channel, affects the energy regulator, informing it about the state of the system.

Feedback called the impact of the results of any process on its course.

If such an impact leads to an increase in the intensity of the process, then the feedback is called positive. If the impact leads to a decrease in the intensity of the process, then the feedback is called negative.

In a self-oscillating system, both positive and negative feedback can be present.

An example of a self-oscillating system is a clock in which the pendulum receives shocks due to the energy of a raised weight or a twisted spring, and these shocks occur at those moments when the pendulum passes through the middle position.

Examples of biological self-oscillatory systems are such organs as the heart and lungs.

1.6. Oscillations of the human body and their registration

The analysis of oscillations created by the human body or its individual parts is widely used in medical practice.

Oscillatory movements of the human body when walking

Walking is a complex periodic locomotor process resulting from the coordinated activity of the skeletal muscles of the trunk and limbs. Analysis of the walking process provides many diagnostic features.

A characteristic feature of walking is the periodicity of the support position with one foot (single support period) or two legs (double support period). Normally, the ratio of these periods is 4:1. When walking, there is a periodic displacement of the center of mass (CM) along the vertical axis (normally by 5 cm) and deviation to the side (normally by 2.5 cm). In this case, the CM moves along a curve, which can be approximately represented by a harmonic function (Fig. 1.8).

Rice. 1.8. Vertical displacement of the CM of the human body during walking

Complex oscillatory movements while maintaining the vertical position of the body.

A person standing vertically experiences complex oscillations of the common center of mass (MCM) and center of pressure (CP) of the feet on the support plane. Based on the analysis of these fluctuations statokinesimetry- a method for assessing a person's ability to maintain an upright posture. By keeping the GCM projection within the coordinates of the boundary of the support area. This method is implemented using a stabilometric analyzer, the main part of which is a stabiloplatform on which the subject is in a vertical position. Oscillations made by the subject's CP while maintaining a vertical posture are transmitted to the stabiloplatform and recorded by special strain gauges. The strain gauge signals are transmitted to the recording device. At the same time, it is recorded statokinesigram - the trajectory of the test subject's movement on a horizontal plane in a two-dimensional coordinate system. According to the harmonic spectrum statokinesigrams it is possible to judge the features of verticalization in the norm and with deviations from it. This method makes it possible to analyze the indicators of statokinetic stability (SCR) of a person.

Mechanical vibrations of the heart

There are various methods for studying the heart, which are based on mechanical periodic processes.

Ballistocardiography(BCG) - a method for studying the mechanical manifestations of cardiac activity, based on the registration of pulse micro-movements of the body, caused by the ejection of blood from the ventricles of the heart into large vessels. This gives rise to the phenomenon returns. The human body is placed on a special movable platform located on a massive fixed table. The platform as a result of recoil comes into a complex oscillatory motion. The dependence of the displacement of the platform with the body on time is called a ballistocardiogram (Fig. 1.9), the analysis of which allows one to judge the movement of blood and the state of cardiac activity.

Apexcardiography(AKG) - a method of graphic registration of low-frequency oscillations of the chest in the area of ​​the apex beat, caused by the work of the heart. Registration of the apexcardiogram is performed, as a rule, on a multichannel electrocardiogram.

Rice. 1.9. Recording a ballistocardiogram

graph using a piezocrystalline sensor, which is a converter of mechanical vibrations into electrical ones. Before recording on the anterior wall of the chest, the point of maximum pulsation (apex beat) is determined by palpation, in which the sensor is fixed. Based on the sensor signals, an apexcardiogram is automatically built. An amplitude analysis of the ACG is carried out - the amplitudes of the curve are compared at different phases of the work of the heart with a maximum deviation from the zero line - the EO segment, taken as 100%. Figure 1.10 shows the apexcardiogram.

Rice. 1.10. Apexcardiogram recording

Kinetocardiography(KKG) - a method of recording low-frequency vibrations of the chest wall, caused by cardiac activity. The kinetocardiogram differs from the apexcardiogram: the former captures the record absolute movements chest wall in space, the second registers the fluctuations of the intercostal spaces relative to the ribs. This method determines the displacement (KKG x), the speed of movement (KKG v) as well as the acceleration (KKG a) for chest oscillations. Figure 1.11 shows a comparison of various kinetocardiograms.

Rice. 1.11. Recording kinetocardiograms of displacement (x), speed (v), acceleration (a)

Dynamocardiography(DKG) - a method for assessing the movement of the center of gravity of the chest. Dynamocardiograph allows you to register the forces acting from the human chest. To record a dynamocardiogram, the patient is positioned on the table lying on his back. Under the chest there is a perceiving device, which consists of two rigid metal plates measuring 30x30 cm, between which there are elastic elements with strain gauges mounted on them. Periodically changing in magnitude and place of application, the load acting on the receiving device is composed of three components: 1) a constant component - the mass of the chest; 2) variable - mechanical effect of respiratory movements; 3) variable - mechanical processes accompanying cardiac contraction.

The recording of the dynamocardiogram is carried out with the patient holding their breath in two directions: relative to the longitudinal and transverse axes of the receiving device. Comparison of various dynamocardiograms is shown in fig. 1.12.

Seismocardiography is based on the registration of mechanical vibrations of the human body caused by the work of the heart. In this method, using sensors installed in the region of the base of the xiphoid process, a cardiac impulse is recorded due to the mechanical activity of the heart during the period of contraction. At the same time, processes occur associated with the activity of tissue mechanoreceptors of the vascular bed, which are activated when the volume of circulating blood decreases. The seismocardiosignal forms the shape of the sternum oscillations.

Rice. 1.12. Recording of normal longitudinal (a) and transverse (b) dynamocardiograms

Vibration

The widespread introduction of various machines and mechanisms into human life increases labor productivity. However, the work of many mechanisms is associated with the occurrence of vibrations that are transmitted to a person and have a harmful effect on him.

Vibration- forced oscillations of the body, in which either the whole body oscillates as a whole, or its separate parts oscillate with different amplitudes and frequencies.

A person constantly experiences various kinds of vibrational effects in transport, at work, at home. Vibrations that have arisen in any place of the body (for example, the hand of a worker holding a jackhammer) propagate throughout the body in the form of elastic waves. These waves cause variable deformations of various types in the tissues of the body (compression, tension, shear, bending). The effect of vibrations on a person is due to many factors that characterize vibrations: frequency (frequency spectrum, fundamental frequency), amplitude, speed and acceleration of an oscillating point, energy of oscillatory processes.

Prolonged exposure to vibrations causes persistent disturbances in normal physiological functions in the body. "Vibration sickness" may occur. This disease leads to a number of serious disorders in the human body.

The influence that vibrations have on the body depends on the intensity, frequency, duration of vibrations, the place of their application and direction in relation to the body, posture, as well as on the state of the person and his individual characteristics.

Fluctuations with a frequency of 3-5 Hz cause reactions of the vestibular apparatus, vascular disorders. At frequencies of 3-15 Hz, disorders associated with resonant vibrations of individual organs (liver, stomach, head) and the body as a whole are observed. Fluctuations with frequencies of 11-45 Hz cause blurred vision, nausea, and vomiting. At frequencies exceeding 45 Hz, damage to the vessels of the brain, impaired blood circulation, etc. occur. Figure 1.13 shows the vibration frequency ranges that have a harmful effect on a person and his organ systems.

Rice. 1.13. The frequency ranges of the harmful effects of vibration on humans

At the same time, in some cases, vibrations are used in medicine. For example, using a special vibrator, the dentist prepares an amalgam. The use of high-frequency vibration devices allows drilling a hole of complex shape in the tooth.

Vibration is also used in massage. With manual massage, the massaged tissues are brought into oscillatory motion with the help of the massage therapist's hands. With hardware massage, vibrators are used, in which tips of various shapes are used to transmit oscillatory movements to the body. Vibrating devices are divided into devices for general vibration, causing shaking of the whole body (vibrating "chair", "bed", "platform", etc.), and devices for local vibration impact on individual parts of the body.

Mechanotherapy

In physiotherapy exercises (LFK), simulators are used, on which oscillatory movements are carried out various parts human body. They are used in mechanotherapy - form of exercise therapy, one of the tasks of which is the implementation of dosed, rhythmically repeated physical exercises for the purpose of training or restoring mobility in the joints on pendulum-type devices. The basis of these devices is balancing (from fr. balancer- swing, balance) a pendulum, which is a two-arm lever that performs oscillatory (rocking) movements around a fixed axis.

1.7. Basic concepts and formulas

Table continuation

Table continuation

End of table

1.8. Tasks

1. Give examples of oscillatory systems in humans.

2. In an adult, the heart makes 70 contractions per minute. Determine: a) the frequency of contractions; b) the number of cuts in 50 years

Answer: a) 1.17 Hz; b) 1.84x10 9 .

3. What length must a mathematical pendulum have in order for its period of oscillation to be equal to 1 second?

4. A thin straight homogeneous rod 1 m long is suspended by its end on an axis. Determine: a) what is the period of its oscillations (small)? b) what is the length of a mathematical pendulum with the same period of oscillation?

5. A body with a mass of 1 kg oscillates according to the law x = 0.42 cos (7.40t), where t is measured in seconds, and x is measured in meters. Find: a) amplitude; b) frequency; c) total energy; d) kinetic and potential energies at x = 0.16 m.

6. Estimate the speed at which a person walks with a stride length l= 0.65 m. Leg length L = 0.8 m; the center of gravity is at a distance H = 0.5 m from the foot. For the moment of inertia of the leg relative to the hip joint, use the formula I = 0.2mL 2 .

7. How can you determine the mass of a small body aboard a space station if you have a clock, a spring, and a set of weights at your disposal?

8. The amplitude of damped oscillations decreases in 10 oscillations by 1/10 of its original value. Oscillation period T = 0.4 s. Determine the logarithmic decrement and damping factor.

fluctuations- These are movements or processes that repeat exactly or approximately at certain intervals of time.

Mechanical oscillations - fluctuations in mechanical quantities (displacement, speed, acceleration, pressure, etc.).

Mechanical vibrations (depending on the nature of the forces) are:

free;

forced;

self-oscillations.

Free called vibrations that occur when a single action of an external force (the initial message of energy) and in the absence of external influences on the oscillatory system.

Free (or own)- these are oscillations in the system under the action of internal forces, after the system is taken out of equilibrium (in real conditions, free oscillations are always damped).

Conditions for the occurrence of free oscillations

1. The oscillatory system must have a position of stable equilibrium.

2. When the system is taken out of equilibrium, a resultant force must arise that returns the system to its original position

3. Forces of friction (resistance) are very small.

Forced vibrations- fluctuations occurring under the influence of external forces that change over time.

Self-oscillations- undamped oscillations in the system, supported by internal energy sources in the absence of an external variable force.

The frequency and amplitude of self-oscillations is determined by the properties of the oscillatory system itself.

Self-oscillations differ from free oscillations in that the amplitude is independent of time and of the initial action that excites the process of oscillations.

Self-oscillating system consists of: oscillating system; energy source; feedback device that regulates the flow of energy from an internal energy source into an oscillatory system.

The energy coming from the source in a period is equal to the energy lost by the oscillatory system in the same time.

Mechanical vibrations are divided into:

fading;

undamped.

damped vibrations- fluctuations, the energy of which decreases with time.

Characteristics of the oscillatory movement:

permanent:

amplitude (A)

period (T)

frequency()

The greatest (in absolute value) deviation of an oscillating body from the equilibrium position is called vibration amplitude. Typically, the amplitude is denoted by the letter A.

The time interval during which the body completes one complete oscillation is called period of oscillation.

The period of oscillation is usually denoted by the letter T and in SI is measured in seconds (s).

The number of oscillations per unit time is called oscillation frequency.

The frequency is denoted by the letter v (“nu”). The unit of frequency is one oscillation per second. This unit is named hertz (Hz) in honor of the German scientist Heinrich Hertz.

the oscillation period T and the oscillation frequency v are related by the following relationship:

T=1/ or =1/T.

Cyclic (circular) frequency ω is the number of oscillations in 2π seconds

Harmonic vibrations- mechanical vibrations that occur under the action of a force proportional to the displacement and directed opposite to it. Harmonic vibrations are made according to the law of sine or cosine.

Let the material point perform harmonic oscillations.

The equation of harmonic oscillations has the form:

a - acceleration V - speed q - charge A - amplitude t - time

Themes USE codifier: harmonic vibrations; amplitude, period, frequency, phase of oscillations; free vibrations, forced vibrations, resonance.

fluctuations are changes in the state of the system that repeat over time. The concept of oscillations covers a very wide range of phenomena.

fluctuations mechanical systems, or mechanical vibrations- this is a mechanical movement of a body or a system of bodies, which has a repeatability in time and occurs in the vicinity of the equilibrium position. equilibrium position This is the state of the system in which it can remain for an arbitrarily long time without experiencing external influences.

For example, if the pendulum is deflected and released, then oscillations will begin. The equilibrium position is the position of the pendulum in the absence of deflection. In this position, the pendulum, if left untouched, can remain indefinitely. When the pendulum oscillates, it passes the equilibrium position many times.

Immediately after the deflected pendulum was released, it began to move, passed the equilibrium position, reached the opposite extreme position, stopped for a moment in it, moved in the opposite direction, passed the equilibrium position again and returned back. One thing happened full swing. This process will then be repeated periodically.

Amplitude of body oscillations is the magnitude of its greatest deviation from the equilibrium position.

Oscillation period is the time for one complete oscillation. We can say that for the period the body travels a path of four amplitudes.

Oscillation frequency is the reciprocal of the period: . Frequency is measured in hertz (Hz) and indicates how many complete oscillations occur in one second.

Harmonic vibrations.

We will assume that the position of the oscillating body is determined by a single coordinate . The value corresponds to the equilibrium position. The main task of mechanics in this case is to find a function that gives the coordinate of the body at any time.

For the mathematical description of oscillations, it is natural to use periodic functions. There are many such functions, but two of them - sine and cosine - are the most important. They have many good properties and are closely related to a wide range of physical phenomena.

Since the sine and cosine functions are obtained from each other by shifting the argument by , we can limit ourselves to only one of them. For definiteness, we will use the cosine.

Harmonic vibrations are oscillations in which the coordinate depends on time according to the harmonic law:

(1)

Let us find out the meaning of the quantities included in this formula.

A positive value is the largest coordinate value in absolute value (since the maximum value of the cosine modulus is equal to one), i.e., the largest deviation from the equilibrium position. Therefore - the amplitude of oscillations.

The cosine argument is called phase fluctuations. The value equal to the value of the phase at is called the initial phase. The initial phase corresponds to the initial coordinate of the body: .

The value is called cyclic frequency. Let's find its connection with the oscillation period and frequency. One complete oscillation corresponds to a phase increment equal to radians: , whence

(2)

(3)

The cyclic frequency is measured in rad/s (radians per second).

In accordance with expressions (2) and (3), we obtain two more forms of recording the harmonic law (1) :

The graph of the function (1), which expresses the dependence of the coordinate on time for harmonic oscillations, is shown in fig. one .

The harmonic law of the form (1) is of the most general nature. It answers, for example, situations when two initial actions: deflected by an amount and given it some initial velocity. There are two important special cases where one of these actions was not performed.

Let the pendulum be rejected, but the initial speed was not reported (they were released without the initial speed). It is clear that in this case , so we can put . We get the law of cosine:

The graph of harmonic oscillations in this case is shown in Fig. 2.

Rice. 2. Law of cosine

Let us now assume that the pendulum was not deflected, but the initial velocity was imparted to it from the equilibrium position by a blow. In this case , so you can put . We get the sine law:

The schedule of fluctuations is presented on fig. 3 .

Rice. 3. Law of sine

The equation of harmonic oscillations.

Let's return to the general harmonic law (1) . Let's differentiate this equation:

. (4)

Now we differentiate the resulting equality (4) :

. (5)

Let's compare expression (1) for the coordinate and expression (5) for the acceleration projection. We see that the acceleration projection differs from the coordinate only by the factor :

. (6)

This ratio is called equation of harmonic oscillations. It can also be rewritten in this form:

. (7)

From a mathematical point of view, equation (7) is differential equation. Decisions differential equations serve as functions (and not numbers, as in ordinary algebra).
So, we can prove that:

The solution of equation (7) is any function of the form (1) with arbitrary ;

No other function is a solution to this equation.

In other words, relations (6) , (7) describe harmonic oscillations with a cyclic frequency and only them. Two constants are determined from the initial conditions - by the initial values ​​of the coordinate and velocity.

Spring pendulum.

Spring pendulum is a load fixed on a spring, capable of oscillating in a horizontal or vertical direction.

Let's find the period of small horizontal oscillations of the spring pendulum (Fig. 4). Oscillations will be small if the magnitude of the deformation of the spring is much less than its dimensions. For small deformations, we can use Hooke's law. This will cause the oscillations to be harmonic.

We neglect friction. The mass has a mass and the spring constant is .

The coordinate corresponds to the equilibrium position in which the spring is not deformed. Therefore, the magnitude of the spring deformation is equal to the modulus of the load coordinate.

Rice. 4. Spring pendulum

In the horizontal direction, only the elastic force from the spring acts on the load. Newton's second law for the load in the projection on the axis is:

. (8)

If (the load is shifted to the right, as in the figure), then the elastic force is directed in the opposite direction, and . Conversely, if , then . The signs and are opposite all the time, so Hooke's law can be written as follows:

Then relation (8) takes the form:

We have obtained an equation of harmonic oscillations of the form (6) , in which

The cyclic frequency of oscillation of a spring pendulum is thus equal to:

. (9)

From here and from the ratio we find the period of horizontal oscillations of the spring pendulum:

. (10)

If you hang a weight on a spring, you get a spring pendulum that oscillates in the vertical direction. It can be shown that in this case the formula (10) is also valid for the oscillation period.

Mathematical pendulum.

Mathematical pendulum - this is a small body suspended on a weightless inextensible thread (Fig. 5). A mathematical pendulum can oscillate in a vertical plane in the field of gravity.

Rice. 5. Mathematical pendulum

Let us find the period of small oscillations of the mathematical pendulum. The thread length is . Air resistance is neglected.

Let's write down Newton's second law for the pendulum:

and project it onto the axis :

If the pendulum occupies a position as in the figure (i.e.), then:

If the pendulum is on the other side of the equilibrium position (i.e.), then:

So, for any position of the pendulum we have:

. (11)

When the pendulum is at rest in the equilibrium position, the equality is fulfilled. For small oscillations, when the deviations of the pendulum from the equilibrium position are small (compared to the length of the thread), the approximate equality is fulfilled. Let's use it in formula (11):

This is an equation of harmonic oscillations of the form (6) in which

Therefore, the cyclic oscillation frequency of a mathematical pendulum is equal to:

. (12)

Hence the period of oscillation of the mathematical pendulum:

. (13)

Please note that formula (13) does not include the mass of the load. Unlike a spring pendulum, the period of oscillation of a mathematical pendulum does not depend on its mass.

Free and forced vibrations.

The system is said to free vibrations, if it is once taken out of the equilibrium position and subsequently left to itself. No periodic external
At the same time, the system does not experience any impacts, and there are no internal sources of energy supporting oscillations in the system.

The vibrations of the spring and mathematical pendulums are examples of free vibrations.

The frequency at which free vibrations occur is called natural frequency oscillatory system. So, formulas (9) and (12) give natural (cyclic) oscillation frequencies of spring and mathematical pendulums.

In an idealized situation in the absence of friction, free oscillations are undamped, i.e., they have a constant amplitude and last indefinitely. In real oscillatory systems, friction is always present, so free oscillations gradually damp out (Fig. 6).

Forced vibrations- these are oscillations made by the system under the influence of an external force, periodically changing in time (the so-called driving force).

Let us assume that the natural oscillation frequency of the system is , and the driving force depends on time according to the harmonic law:

For some time, forced oscillations are established: the system performs a complex movement, which is a superposition of forced and free oscillations. Free oscillations gradually damp out, and in the steady state the system performs forced oscillations, which also turn out to be harmonic. The frequency of steady forced oscillations coincides with the frequency
driving force (an external force, as it were, imposes its frequency on the system).

The amplitude of steady-state forced oscillations depends on the frequency of the driving force. The graph of this dependence is shown in Fig. 7.

Rice. 7. Resonance

We see that resonance occurs near the frequency - the phenomenon of an increase in the amplitude of forced oscillations. The resonant frequency is approximately equal to the natural oscillation frequency of the system: , and this equality is the more accurate, the less friction in the system. In the absence of friction, the resonant frequency coincides with the natural oscillation frequency, , and the oscillation amplitude increases to infinity at .

Mechanical vibrations are called movements that are exactly or approximately exactly repeated at certain intervals of time. It is characteristic of oscillations that an oscillating body, for example, a pendulum, alternately shifts either in one direction or in the other. When the body rotates, the movement also periodically repeats, but displacements in opposite directions relative to the equilibrium position do not occur. Vibrational and rotational movement are caused by forces, which, as a rule, depend differently on the distances between the bodies.
§1.1. CLASSIFICATION OF OSCILLATIONS
According to the nature of the physical processes in the system that cause oscillatory movements, there are three main types of oscillations: free, forced and self-oscillations.
Free vibrations

The simplest type of vibrations are free vibrations. Free vibrations arise in the system under the action of internal forces after the system is taken out of equilibrium. Such vibrations are made by a load suspended on a spring igls. 1.1), a ball on a thread (pendulum) (Fig. 1.2), etc.
These systems have a stable position of equilibrium, in which the forces acting on the body are mutually balanced
-»
sheny. The force of gravity F acting on the ball is balanced
-»
on or by the elastic force of the stretched spring F0 (Fig. 1.3),
-»
or the tension force of the pendulum thread FQ (Fig. 1.4). When the system is removed from the equilibrium position, the action begins
Rice. 1.3
Rice. 1.4
O
vat forces directed to this position. As a result, fluctuations occur.
He
Let us consider in more detail why oscillations occur, for example, of a ball suspended on a spring. If you move the ball down so that the length of the spring increases by x (Fig. 1.5), then an additional force will begin to act on the ball
F..
X
Rice. 1.5
elasticity ґ , the modulus of which is proportional according to Hooke's law to the elongation of the spring. This force is directed upward, and under its influence, the ball will begin to move upward with acceleration, gradually increasing its speed. The force will decrease as the spring contracts. At the moment when the ball reaches the equilibrium position, the sum of all forces acting on it will become equal to zero. Consequently, the acceleration of the ball according to Newton's second law will become equal to zero.
But by this moment the speed of the ball will already reach a certain value. Therefore, without stopping in the equilibrium position, it will continue to rise up by inertia. In this case, the spring is compressed, and as a result, a force appears that is already directed downward and slows down the movement of the ball (Fig. 1.6). This force, and hence the downward acceleration, increase in direct proportion to the absolute value of the displacement x of the ball relative to the equilibrium position. The speed decreases until it vanishes at the highest point. After that, the ball with acceleration
W
X
Of
will start moving down. As x decreases, the modulus of the force Fy decreases and again vanishes in the equilibrium position. But the ball has already managed to pick up speed by this moment and continues to move down. This movement leads to further stretching of the spring and to the appearance of an upward force. The movement of the ball is slowed down to a complete stop in the lowest position, after which the whole process is repeated from the beginning.
If there were no friction, then the movement of the ball would never stop.
Rice. 1.6
However, there is friction, and the friction force, both when the ball moves up and when moving down, is always directed against the speed. It slows down the movement of the ball, and therefore the range of its oscillations gradually decreases until the movement stops. With low friction, damping becomes noticeable only after the ball has made many oscillations. And if you are interested in the movement of the ball over a not very long time interval, then the damping of its oscillations can be neglected. In this case, the influence of the friction force on the motion can be ignored.
If the friction force is large, then its action cannot be neglected even for small time intervals. Dip the ball on the spring into a glass with a viscous liquid, such as glycerin. If the spring is soft enough, then the unbalanced ball will not oscillate at all. Under the action of the elastic force, he will simply return to the equilibrium position, but he will not rise higher; due to the action of the friction force, its velocity in the equilibrium position will be practically equal to zero.
Now we can figure out what is essential for free vibrations to arise in the system. Two conditions must be met. First, when the body is removed from the equilibrium position, a force must arise in the system, directed towards the equilibrium position, and therefore, tending to return the body to the equilibrium position. This is exactly how the elastic force of the spring and the force of gravity act in the system we have considered: both when the ball moves up and when it moves down, the resulting force is directed towards the equilibrium position. Secondly, the friction in the system must be sufficiently small, otherwise the oscillations will quickly die out or even not arise. Continuous oscillations are possible only in the absence of friction.
Both conditions are quite general, valid for any system in which free oscillations can occur. Check it out for yourself on another simple system - the pendulum. At the same time, it must be borne in mind that the ball on the thread will be a pendulum only if the force of gravity acts on it. The globe that creates this force enters into an oscillatory system, which for brevity we simply call a pendulum.
Forced vibrations
Oscillations made by bodies under the action of external periodically changing forces are called forced.
Such oscillations will, for example, be made by a book on the table if we begin to move it back and forth with our hand. The vibrations of the book in this case are caused by the action of a force from the side of the hand, which changes in magnitude and direction. Forced vibrations are also vibrations of pistons in the cylinders of an internal combustion engine, sewing machine needles, etc. Of particular interest, as we will see later, are forced vibrations in a system capable of performing free vibrations.
Self-oscillations
Most complex view oscillations are self-oscillations. Self-oscillations are called undamped oscillations that can exist in a system without external periodic forces acting on it. To do this, the system must have its own source of energy. Due to the energy of the source, the oscillations do not dampen, despite the action of friction forces. The most well-known self-oscillatory system is a clock with a pendulum or balancer. We will consider self-oscillations at the end of the third chapter.